Statistics 1601
ASSIGNMENT 1: CHAPTER 1 (70 points)
All problems taken from Introduction to the Practice of Statistics, Fifth Edition by David S. Moore and George P. McCabe.
1.7 (2 points) Is driving becoming more dangerous? Traffic deaths declined for years, bottoming out at 39,250 killed in 1992, then began to increase again. In 2002, 42,815 people died in traffic accidents. But more vehicles drove more miles in 2002 than in 1992. In fact, the government says that motor vehicles traveled 2247 billion miles in 1992 and 2830 billion miles in 2002. Reports on transportation use deaths per 100 million miles as a measure of risk. Compare the rates for 1992 and 2002. What do you conclude?
ANSWER:
1.18 (3 points) The study described in the previous exercise also measured the fasting plasma glucose of 16 diabetics who were given individual instruction on diabetes control. Here are the data:
![]()
Make a back-to-back stemplot to compare the class and individual instruction groups. How do the distribution shapes and success in achieving the glucose control goal compare?
ANSWER:
1.20 (2 points) Figure 1.13 (page 30 in 5th edition text; same as Figure 1.11 on page 26 in 4th edition) is a histogram of the lengths of words used in Shakespeare's plays. Because there are so many words in the plays, we use a histogram of percents. What is the overall shape of this distribution? What does this shape say about word lengths in Shakespeare? Do you expect other authors to have word length distributions of the same general shape? Why?
ANSWER:
1.21 (2 points) Jeanna plans to attend college in her home state of Massachusetts. She looks up the tuition and fees for the 2003-2004 academic year for all 56 four-year colleges in Massachusetts (omitting art schools and other special colleges). Figure 1.14 is a histogram of the data. For state schools, Jeanna used the in-state tuition. What is the most important aspect of the overall pattern of this distribution? Why do you think this pattern appears?
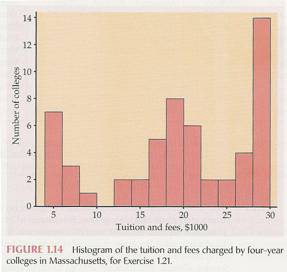
ANSWER:
1.28 (6 points) "Recruitment," the addition of new members to a fish population, is an important measure of the health of ocean ecosystems. Here are data on the recruitment of rock sole in the Bering Sea between 1973 and 2000:
|
Year |
Recruitment (millions) |
Year |
Recruitment (millions) |
Year |
Recruitment (millions) |
Year |
Recruitment (millions) |
|
1973 |
173 |
1980 |
1411 |
1987 |
4700 |
1994 |
505 |
|
1974 |
234 |
1981 |
1431 |
1988 |
1702 |
1995 |
304 |
|
1975 |
616 |
1982 |
1250 |
1989 |
1119 |
1996 |
425 |
|
1976 |
344 |
1983 |
2246 |
1990 |
2407 |
1997 |
214 |
|
1977 |
515 |
1984 |
1793 |
1991 |
1049 |
1998 |
385 |
|
1978 |
576 |
1985 |
1793 |
1992 |
505 |
1999 |
445 |
|
1979 |
727 |
1986 |
2809 |
1993 |
998 |
2000 |
676 |
(a) (3 points) Make a graph to display the distribution of rock sole recruitment, then describe the pattern and any striking deviations that you see.
ANSWER:
(b) (3 points) Make a time plot of recruitment and describe its pattern. As is often the case with time series data, a time plot is needed to understand what is happening.
ANSWER:
1.34 (4 points) In 1798 the English scientist Henry Cavendish measured the density of the earth by careful work with a torsion balance. The variable recorded was the density of the earth as a multiple of the density of water. Here are Cavendish's 29 measurements:
|
Present these measurements graphically by either a stemplot or a histogram and explain the reason for your choice. Then briefly discuss the main features of the distribution. In particular, what is your estimate of the density of the earth based on these measurements?
ANSWER:
1.35 (4 points) Do women study more than men? We asked the students in a large first-year college class how many minutes they studied on a typical weeknight. Here are the responses of random samples of 30 women and 30 men from the class:
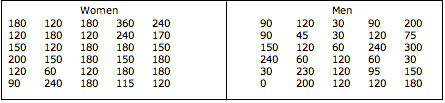 |
(a) (1 point) Examine the data. Why are you not surprised that most responses are multiples of 10 minutes? We eliminated one student who claimed to study 30,000 minutes per night. Are there any other responses you consider suspicious?
ANSWER:
(b) (3 points) Make a back-to-back stemplot of these data. Report the approximate midpoints of both groups. Does it appear that women study more than men (or at least claim that they do)?
ANSWER:
1.58 (6 points) Different varieties of the tropical flower Heliconia are fertilized by different species of hummingbirds. Over time, the lengths of the flowers and the form of the hummingbirds' beaks have evolved to match each other. Here are data on the lengths in millimeters of three varieties of these flowers on the island of Dominica:
|
Make boxplots to compare the three distributions. Report the five-number summaries along with your graph. What are the most important differences among the three varieties of flower?
ANSWER:
1.59 (6 points) The biologists who collected the flower length
data in the previous exercise compared the three Heliconia varieties
using statistical methods based on ![]() and s.
and s.
(a) (2 points) Find ![]() and s for each variety.
and s for each variety.
ANSWER:
(b) (4 points) Make a stemplot of each set of
flower lengths. Do the distributions appear suitable for the use
of ![]() and s as summaries?
and s as summaries?
ANSWER:
1.60 (5 points) "Conservationists have despaired over destruction of tropical rainforest by logging, clearing, and burning." These words began a report on a statistical study of the effects of logging in Borneo. Researches compared forest plots that had never been logged (Group 1) with similar plots nearby that had been logged 1 year earlier (Group 2) and 8 years earlier (Group 3). All plots were 0.1 hectare in area. Here are the counts of trees for plots in each group:
|
Give a complete comparison
of the three distributions, using both graphs and numerical summaries. To
what extent has logging affected the count of trees? The researchers
used an analysis based on ![]() and s. Explain why this is reasonably well justified.
and s. Explain why this is reasonably well justified.
ANSWER:
1.89 (2 points) The heights of women aged 20 to 29 are approximately normal with mean 64 inches and standard deviation 2.7 inches. Men the same age have mean height 69.3 inches with standard deviation 2.8 inches. What are the z-scores for a woman 6 feet tall and a man 6 feet tall? What information do the z-scores give that the actual heights do not?
ANSWER:
1.93 (8 points) Using either Table A or your calculator or software, find the proportion of observations from a standard normal distribution for each of the following events. In each case, sketch a standard normal curve and shade the area representing the proportion.
(a) (2 points) Z ² -2 (this is a cumulative proportion)
ANSWER:
(b) (2 points) Z ³ -2
ANSWER:
(c) (2 points) Z > 1.67
ANSWER:
(d) (2 points) -2 < Z < 1.67
ANSWER:
There are two major tests of readiness for college, the ACT and the SAT. ACT scores are reported on a scale from 1 to 36. The distribution of ACT scores for more than 1 million students in a recent high school graduating class was roughly normal with mean m = 20.8 and standard deviation s = 4.8. SAT scores are reported on a scale from 400 to 1600. The SAT scores for 1.4 million students in the same graduating class were roughly normal with mean m = 1026 and standard deviation s = 209. Exercises 1.98 to 1.109 are based on this information.
1.106 (2 points) What SAT scores make up the top 10% of all scores?
ANSWER:
1.110 (4 points) Too much cholesterol in the blood increases the risk of heart disease. Young women are generally less afflicted with high cholesterol than other groups. The cholesterol levels for women aged 20 to 34 follow an approximately normal distribution with mean 185 milligrams per deciliter (mg/dl) and standard deviation 39 mg/dl.
(a) (2 points) Cholesterol levels above 240 mg/dl demand medical attention. What percent of young women have levels above 240 mg/dl?
ANSWER:
(b) (2 points) Levels above 200 mg/dl are considered borderline high. What percent of young women have blood cholesterol between 200 and 240 mg/dl?
ANSWER:
1.111 (4 points) Middle-aged men are more susceptible to high cholesterol than the young women of Exercise 1.110. The blood cholesterol levels of men aged 55 to 64 are approximately normal with mean 222 mg/dl and standard deviation 37 mg/dl. What percent of these men have high cholesterol (levels above 240 mg/dl)? What percent have borderline high cholesterol (between 200 and 240 mg/dl)?
ANSWER:
1.113 (4 points) Changing the mean of a normal distribution by a moderate amount can greatly change the percent of observations in the tails. Suppose that a college is looking for applicants with SAT math scores 750 and above.
(a) (2 points) In 2003, the scores of men on the math SAT followed a normal distribution with mean 537 and standard deviation 116. What percent of men scored 750 or better?
ANSWER:
(b) (2 points) Women's scores that year had a normal distribution with mean 503 and standard deviation 110. What percent of women scored 750 or better? You see that the percent of men above 750 is more than two and one-half times the percent of women with such high scores.
ANSWER:
1.115 (6 points) The length of human pregnancies from conception to birth varies according to a distribution that is approximately normal with mean 266 days and standard deviation 16 days.
(a) (2 points) What percent of pregnancies last less than 240 days (thatÕs about 8 months)?
ANSWER:
(b) (2 points) What percent of pregnancies last between 240 and 270 days (roughly between 8 months and 9 months)?
ANSWER:
(c) (2 points) How long do the longest 20% of pregnancies last?
ANSWER:
-------------------------------------------------------------------------------------
Total:__/70